flying butterfly linear vibration science experiment equipment technology small production diy science popularization teaching aids science and education

义乌市驰购百货贸易商行 2yr.

Main Products:

玩具

Contacts:

汪先生Chat

Mobile:

86-15267379642

WhatsApp:

15267379642

E-mail:

251044035@qq.com

WeChat:

15267379642

Address:

浙江省金华义乌市江东街道108栋5单元,Yiwu,Jinhua City,Zhejiang Province

Description

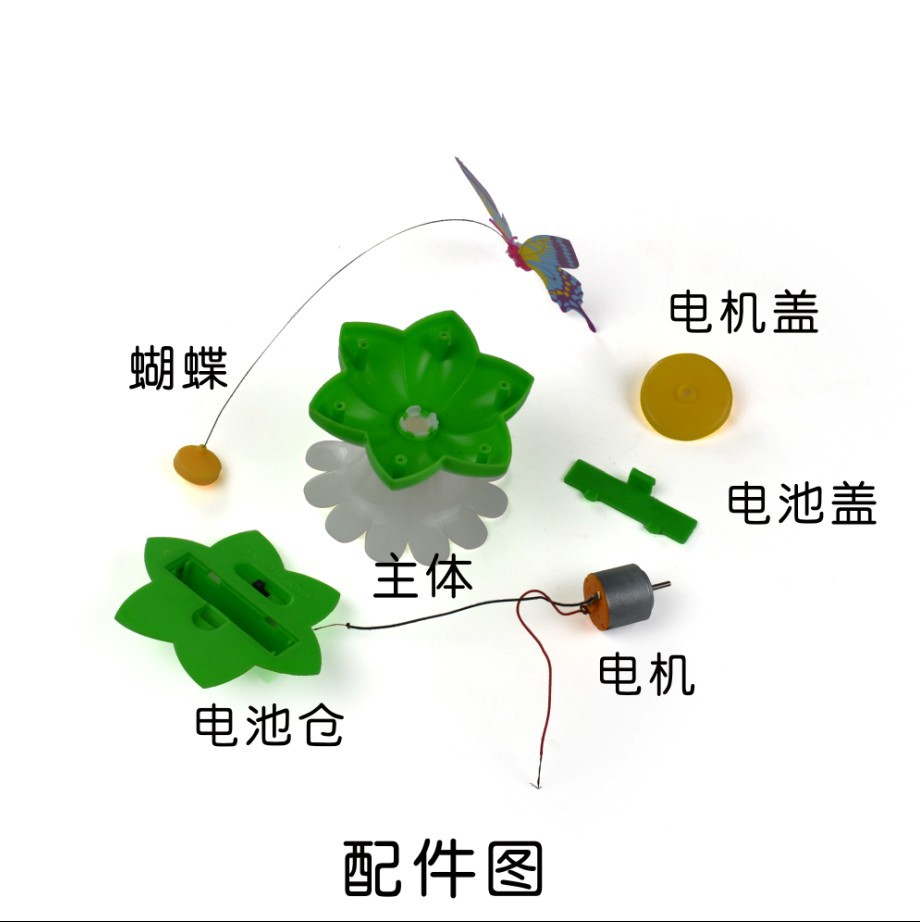
Butterfly Flutter Course Presentation

Linear vibration
The elasticity of the components in the system follows Hooke's Law, and the damping force generated during motion is proportional to the first-order derivative of the generalized velocity (the time derivative of the generalized coordinates).

Linear systems are often abstract models of the slight vibrations of actual systems. Linear vibration systems apply the principle of superposition, which is that if the system response to input x1 is y1, and the system response to input x2 is y2, then the system response to the combined input of x1 and x2 is y1+y2. Based on the principle of superposition, an arbitrary input can be decomposed into a sum of a series of infinitesimal impulses, and then the total response of the system can be obtained. It is also possible to transform a periodic excitation into a sum of a series of harmonic components using Fourier transformation, and to examine the effects of each harmonic component on the system separately. By adding them together, the total response of the system is obtained. Therefore, the response characteristics of constant linear systems can be described by pulse response or frequency response. Pulse response refers to the response of the system to a unit impulse, which characterizes the response characteristics of the system in the time domain. Frequency response refers to the response characteristics of the system to a unit harmonic input, which characterizes the response characteristics of the system in the frequency domain. Both are determined by the Fourier transformation.

Elastic Vibration

The above multi-degree-of-freedom system is an approximate mechanical model of an elastic body. An elastic body has an infinite number of degrees of freedom. Both have a quantitative difference rather than an essential one. Any elastic body has an infinite number of natural frequencies and an infinite number of corresponding principal vibrational modes, and these principal vibrational modes also exist with respect to the orthogonality of mass and stiffness. Any vibration form of an elastic body can also be represented as a linear superposition of the principal vibrational modes. Therefore, for the dynamic response analysis of an elastic body, the principal vibrational mode superposition method is still applicable (see the linear vibration of an elastic body).

Take the vibration of a string for example. Suppose the mass per unit length ismThe fine string, longlTension is set at TAt this time, the natural frequency of the string is determined by the following formula:

f=na/2l （n=1，2，3，...），

In the formula, is the propagation speed of transverse waves along the string direction. The natural frequencies of the string's various orders happen to be half of the fundamental frequency α.lThe integer multiples of this number. This integer multiple relationship leads to the pleasing homophonic structure. In general, the natural frequencies of the order of an elastic body do not exist in this integer multiple relationship.

The first three modes of vibration of the tensioned string are shown in Figure 9, with some nodes present on the main vibration pattern. During the main vibration, no vibration occurs at the nodes. Figure 10 presents several typical modes of vibration for a circular plate with fixed edges, with some node lines composed of circles and diameters depicted on the figure.

The accurate formulation of the problem of elastic vibration can be summarized as a boundary value problem for partial differential equations. However, accurate solutions can only be found in the simplest cases, so for complex elastic vibration problems, one has to resort to approximate methods. The essence of various approximate methods is to change the infinite into the finite, that is, to discretize the continuous system with an infinite number of degrees of freedom into a discrete system with a finite number of degrees of freedom. The widely used discretization methods in engineering analysis fall into two major categories: the finite element method and the modal synthesis method.

Figure 9: Vibration Pattern of a String

Figure 10: Vibration Modes of the Plate

The finite element method abstracts a complex structure into a composite structure composed of a finite number of elements and connected at finite nodes. Each element is an elastic element; the distributed displacement of the element is represented by the interpolation function of the node displacement; and then the distributed parameters of each element are concentrated at each node in a certain format, thereby obtaining the mechanical model of the discrete system.

The modal synthesis method involves decomposing a complex structure into several simpler sub-structures. On the basis of understanding the vibration characteristics of each sub-structure, the method synthesizes these sub-structures into a total structure by taking into account the coordination conditions at the interface. Then, the vibration patterns of the sub-structures are used to predict the vibration patterns of the total structure.

These two methods have both differences and connections, and can be used together. Modal synthesis method can also be effectively combined with experimental determination, forming an analysis method that combines theory with experiment for the vibration problems of large systems.

Company Profile

Chiluo Toy Factory was established in 2007. After ten years of unremitting efforts, our company has gradually matured; Our company is a member of the National Ministry of Education Teaching Equipment Industry Association and a backbone enterprise of Zhejiang Province Teaching Equipment. The company has been awarded the "Heavy Contract and Credit-Keeping" unit for three consecutive years by Yiwu City. In 2014, it was designated by the Zhejiang Provincial Education Bureau as the "Culture and Popularization Experiment Equipment Fixed-point Production Unit."

AiGou Company is a high-tech enterprise that integrates modern scientific research and development, educational equipment, curriculum design, teaching resource integration, and technical services. AiGou, with its high-tech products, is a professional enterprise in the development and production of popular science experiment teaching materials in the country. The products are exported to Europe and the United States.
After years of accumulation, the company has initially reached a certain scale. In terms of technological innovation, the company has professional design personnel engaged in the development of scientific education products and the upgrade and replacement of existing products. In terms of production, management, and service, the company strictly implements the standards of the ISO9001 international quality assurance system. In marketing, the AiGou products have been entered into many provinces and cities in the country and enjoy a good reputation. For many years, it has provided high-quality, advanced, professional series of modern teaching equipment and professional equipment research and development custom services for the educational community. With excellent, high-quality professional talents and perfect after-sales service, it ensures the excellence of product quality and establishes a good corporate image. The scientific education physical series, chemical series, photovoltaic, wind energy, geography, biology, art and other products of Qiquan have successfully won bids in the education bureau tenders many times.

AiGou Company continues to adhere to the business philosophy of 'quality first, service to diligence, honesty in conduct', providing excellent equipment and high-quality service for school teaching and research. We are willing to work diligently with new and old friends at home and abroad, work together, and devote our utmost efforts to education, dedicated to the cause of education.

ChiliBuy ensures that every child can keep up with the times!!!

Update time：20230318111314

	Number		Unit-price		Total
I want to buy：		×	6.5	=	0